Chemical graph theory
Text written by Ivona Traunkar, Faculty of Mathematics, University of Rijeka
Chemical graph theory is the branch of mathematics that applies graph theory to mathematical modeling of chemical molecules. The pioneers of chemical graph theory are Alexandru Balaban, Ante Graovac, Ivan Gutman, Haruo Hosoya, Milan Randić, and Nenad Trinajstić. The basic term of the chemical graph theory is a molecular graph, which represents the structure of the bonds between atoms in the molecules, in terms of the graph theory.
Graph theory is a branch of mathematics derived from the Königsberg bridge problem: can citizens of the Königsberg city (today Kaliningrad, Russia) walk through the city passing every bridge exactly once?
This problem was solved by famous mathematician Leonhard Euler in 1736, and his solution to this problem is considered as the first theorem of the graph theory.

A graph is an ordered pair G=(V, E), where V is a set of vertices, and E is a collection of ordered pairs of vertices (v1,v2). We say that v1 and v2 are adjacent, and the number of neighbors of a vertex v is called the degree of a vertex v.
Euler formed a graph whose vertices are parts of the city divided by bridges, such that two vertices are adjacent if and only if there is a bridge that connects them. Using this graph, he proved that the described walk of the city is not possible.
In the chemical graph theory, an important application has a molecular graph: a graph whose vertices are atoms, and edges represent the chemical bonds between atoms in molecules. In our exhibition, we used a molecular graph of a caffeine molecule.
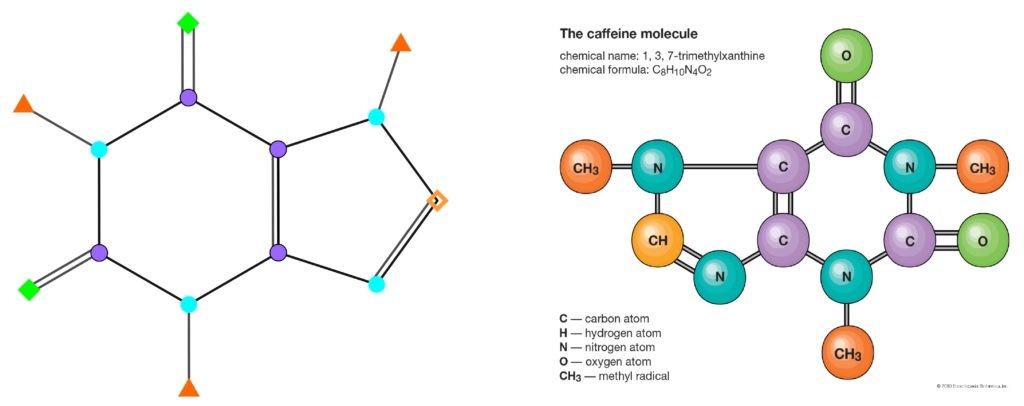
In the chemical graph theory, an important role has molecule descriptors, defined by Todeschini and Consonni as „The molecular descriptor is the final result of a logic and mathematical procedure which transforms chemical information encoded within a symbolic representation of a molecule into a useful number or the result of some standardized experiment”.
We divide them into two main categories: experimental and theoretical molecular descriptors, and we point out topological indices of a graph (also called connection indices) which describe the structure of a molecular graph. Two famous topological indices are the first and second Zagreb index. In 1972, Trinajstić and Gutman developed a formula concerning the total energy of electrons of molecules in which they mention the sum of squares of the degrees of vertices of the molecular graph. Today, this sum is known as the first Zagreb index. The Zagreb indices have been studied extensively due to their numerous applications in the place of existing chemical methods which need more time and increase the costs. More about Zagreb indices you can find at https://www.scirp.org/journal/paperinformation?paperid=95429#ref8