A story about mathematics and crystals
Text written by Bojan Crnković and Vedrana Mikulić Crnković, Faculty of Mathematics, University of Rijeka.
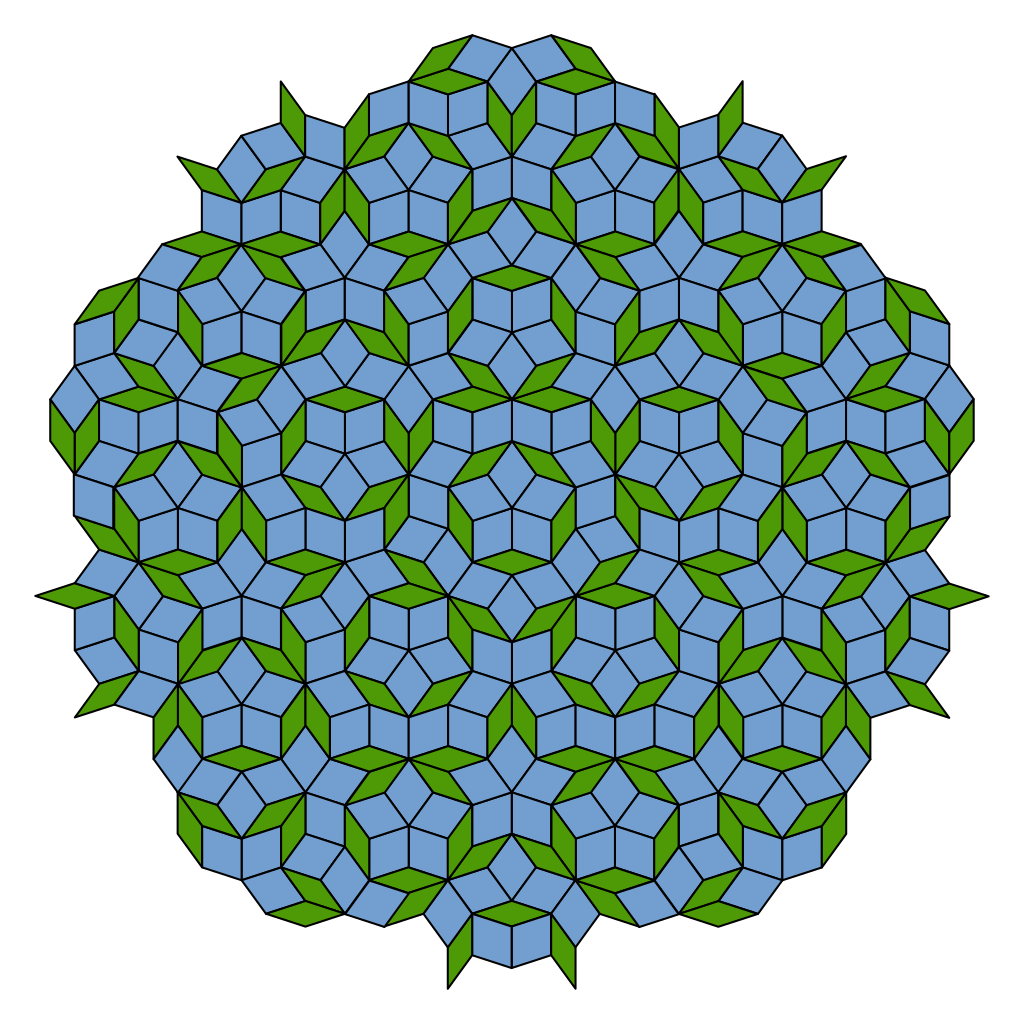
The image shows a combination of tiles inspired by the tilings of the Dutch artist Maurits Cornelis Escher (1898 – 1972) and Penrose tiles with a little help from artificial intelligence (bing/copilot). We have included the image in the exhibition to tell you a short story about the connections between mathematics and crystallography.
The first records of crystals date back to Babylon, ancient India and ancient China, but significant progress in crystallography did not begin until the 17th century. The mathematician and astronomer Johannes Kepler (1571 – 1630) was the first to describe the crystal mathematically. He wondered why snowflakes always had six tips and not five or seven. Crystallography flourished after his observation and his work on hexagonal snow crystals is regarded as the first step towards the discovery of the law of constancy of the angles between the crystal faces. At this time, the geologist Niels Stensen (1638 – 1686) discovered a law that would later become known as Steno’s law of crystallography. The mineralogist René-Just Haüy (1736 – 1790) was the first to notice periodicity in the structure of crystals and is considered the founder of modern crystallography.
A crystal is a geometric body with a regular internal arrangement that is formed through the process of crystallisation. Crystallography is the science of the formation, shape, symmetry, structure and properties of crystals. Each crystal consists of three-dimensionally regularly arranged structural units, whose arrangement of which determines the characteristic properties and shape of the crystal. The structure of the crystal is described by unit or elementary cells, which represent the smallest units of the crystal and contain the smallest possible number of structural units. Symmetrical images of such unit cells in space result in a whole crystal. Another important influence on crystallography was the discovery of X-rays, which made it possible to look into the inner structure of crystals and determine the crystal structure of biologically important molecules.
Crystals in nature are analysed and described by the mathematical theory of groups, i.e. symmetries and crystallographic groups.
In mathematics, the process by which a crystal is formed is called space filling. The filling of two-dimensional space is called tiling of the plane. The tiling of the plane has fascinated many mathematicians throughout history. However, since ancient times, people have used paving stones to decorate their living spaces and create interesting works of art.
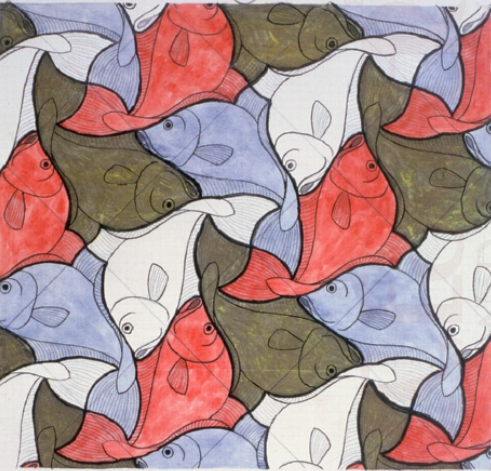
In 1974, British mathematician Roger Penrose created a tiling of a plane in a never-repeating pattern. In 1982, Daniel Shechtman, an Israeli chemist, discovered a metal alloy whose atoms were organised differently than anything ever observed in materials science, confirming that Penrose tiles can occur in nature. An Israeli chemist was awarded the Nobel Prize in Chemistry in 2011 for his discovery of quasicrystals, a type of crystal in which the atoms are arranged in a pattern that follows mathematical rules without the pattern ever repeating itself. Quasicrystals were confirmed in several scientific papers after this revolutionary discovery. Although many thought that quasicrystals were just a scientific hypothesis that could not be realised in nature, nature has once again proved to be an inexhaustible source of inspiration.